Path Sum II

Medium

Given the root of a binary tree and an integer targetSum, return all root-to-leaf paths where the sum of the node values in the path equals targetSum. Each path should be returned as a list of the node values, not node references.

A root-to-leaf path is a path starting from the root and ending at any leaf node. A leaf is a node with no children.

Example 1:

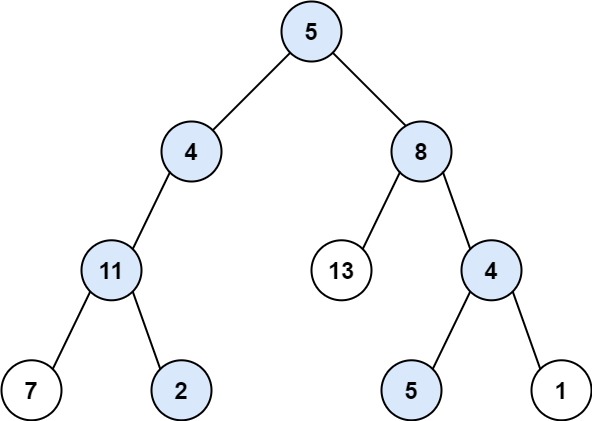
Input: root = [5,4,8,11,null,13,4,7,2,null,null,5,1], targetSum = 22

Output: [[5,4,11,2], [5,8,4,5]]

Explanation: There are two paths whose sum equals targetSum:

5 + 4 + 11 + 2 = 22

5 + 8 + 4 + 5 = 22

Example 2:

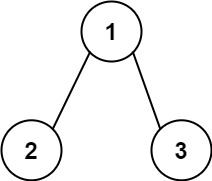
Input: root = [1,2,3], targetSum = 5

Output: []

Example 3:

Input: root = [1,2], targetSum = 0

Output: []

Constraints:

The number of nodes in the tree is in the range [0, 5000].
-1000 <= Node.val <= 1000
-1000 <= targetSum <= 1000

Solution

We wish to fill in the template logic:

is_leaf: when node is a leaf in the tree, and there is no remaining left.
get_edges: the children of the current node (node.left and node.right).
is_valid: an edge (node) is only invalid when it is non-empty (None, null).

In the implementation, we want to check whether node is None first, so that we do not try to get the field of an empty object. On the current node, we calculate the remaining value after adding the current value. Then we check whether the node is a leaf so that path is a root-to-leaf path and whether the remaining value left is 0. If these condition is satisfied, then we have found one solution. If not, we'd have to traverse further until we reach a leaf in the tree (may or may not be a solution).

The below implementation may look different than the template, but essentially one can update and revert path and remaining inside the if-else conditions to gain similarity to the template. We had also left the check of node is None on the outside to accommodate the use of node.val (and to prevent the root being empty).

Implementation

def pathSum(self, root: Optional[TreeNode], targetSum: int) -> List[List[int]]:
    def dfs(node, remaining, path):
        if (node is None): return
        path.append(node.val)     # update path
        remaining -= node.val
        if node.left is None and node.right is None and remaining == 0: # is_leaf
            paths.append(path[:])
        else:     # edges = [node.left, node.right]
            dfs(node.left, remaining, path)
            dfs(node.right, remaining, path)
        path.pop()                # revert path

    paths = []
    dfs(root, targetSum, [])
    return paths

Quick Interview Experience

Help others by sharing your interview experience

Have you seen this problem before?

Ready to land your dream job?

Unlock your dream job with a 5-minute evaluator for a personalized learning plan!

Start Evaluator

Discover Your Strengths and Weaknesses: Take Our 5-Minute Quiz to Tailor Your Study Plan:

What's the output of running the following function using input [30, 20, 10, 100, 33, 12]?

1def fun(arr: List[int]) -> List[int]:
2    import heapq
3    heapq.heapify(arr)
4    res = []
5    for i in range(3):
6        res.append(heapq.heappop(arr))
7    return res
8

1public static int[] fun(int[] arr) {
2    int[] res = new int[3];
3    PriorityQueue<Integer> heap = new PriorityQueue<>();
4    for (int i = 0; i < arr.length; i++) {
5        heap.add(arr[i]);
6    }
7    for (int i = 0; i < 3; i++) {
8        res[i] = heap.poll();
9    }
10    return res;
11}
12

1class HeapItem {
2    constructor(item, priority = item) {
3        this.item = item;
4        this.priority = priority;
5    }
6}
7
8class MinHeap {
9    constructor() {
10        this.heap = [];
11    }
12
13    push(node) {
14        // insert the new node at the end of the heap array
15        this.heap.push(node);
16        // find the correct position for the new node
17        this.bubble_up();
18    }
19
20    bubble_up() {
21        let index = this.heap.length - 1;
22
23        while (index > 0) {
24            const element = this.heap[index];
25            const parentIndex = Math.floor((index - 1) / 2);
26            const parent = this.heap[parentIndex];
27
28            if (parent.priority <= element.priority) break;
29            // if the parent is bigger than the child then swap the parent and child
30            this.heap[index] = parent;
31            this.heap[parentIndex] = element;
32            index = parentIndex;
33        }
34    }
35
36    pop() {
37        const min = this.heap[0];
38        this.heap[0] = this.heap[this.size() - 1];
39        this.heap.pop();
40        this.bubble_down();
41        return min;
42    }
43
44    bubble_down() {
45        let index = 0;
46        let min = index;
47        const n = this.heap.length;
48
49        while (index < n) {
50            const left = 2 * index + 1;
51            const right = left + 1;
52
53            if (left < n && this.heap[left].priority < this.heap[min].priority) {
54                min = left;
55            }
56            if (right < n && this.heap[right].priority < this.heap[min].priority) {
57                min = right;
58            }
59            if (min === index) break;
60            [this.heap[min], this.heap[index]] = [this.heap[index], this.heap[min]];
61            index = min;
62        }
63    }
64
65    peek() {
66        return this.heap[0];
67    }
68
69    size() {
70        return this.heap.length;
71    }
72}
73
74function fun(arr) {
75    const heap = new MinHeap();
76    for (const x of arr) {
77        heap.push(new HeapItem(x));
78    }
79    const res = [];
80    for (let i = 0; i < 3; i++) {
81        res.push(heap.pop().item);
82    }
83    return res;
84}
85

[30, 20, 10]

[100, 33, 30]

[10, 12, 20]

I don't know

Path Sum II

Solution

Implementation

Ready to land your dream job?

Unlock your dream job with a 5-minute evaluator for a personalized learning plan!

Recommended Readings