Pacific Atlantic Water Flow

There is an m x n rectangular island that borders both the Pacific Ocean and Atlantic Ocean. The Pacific Ocean touches the island's left and top edges, and the Atlantic Ocean touches the island's right and bottom edges.

The island is partitioned into a grid of square cells. You are given an m x n integer matrix heights where heights[r][c] represents the height above sea level of the cell at coordinate (r, c).

The island receives a lot of rain, and the rain water can flow to neighboring cells directly north, south, east, and west if the neighboring cell's height is less than or equal to the current cell's height. Water can flow from any cell adjacent to an ocean into the ocean.

Return a 2D list of grid coordinates result where result[i] = [ri, ci] denotes that rain water can flow from cell (ri, ci) to both the Pacific and Atlantic oceans.

Example 1:

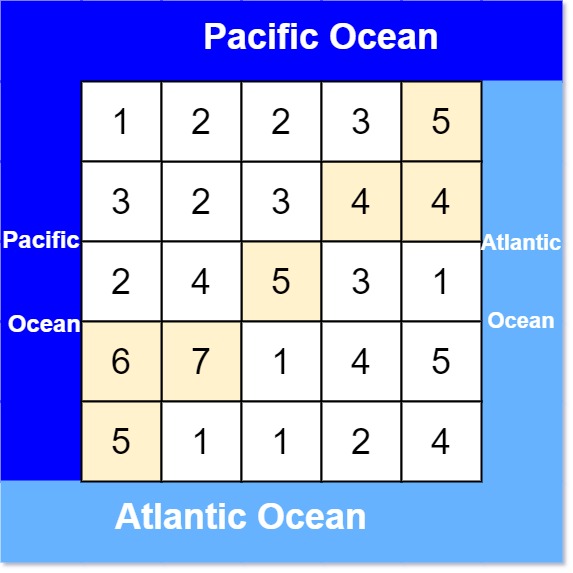
Input: heights = [[1,2,2,3,5],[3,2,3,4,4],[2,4,5,3,1],[6,7,1,4,5],[5,1,1,2,4]]
Output: [[0,4],[1,3],[1,4],[2,2],[3,0],[3,1],[4,0]]
Explanation: The following cells can flow to the Pacific and Atlantic oceans, as shown below:\

1[0,4]: [0,4] -> Pacific Ocean 
2       [0,4] -> Atlantic Ocean
3[1,3]: [1,3] -> [0,3] -> Pacific Ocean 
4       [1,3] -> [1,4] -> Atlantic Ocean
5[1,4]: [1,4] -> [1,3] -> [0,3] -> Pacific Ocean 
6       [1,4] -> Atlantic Ocean
7[2,2]: [2,2] -> [1,2] -> [0,2] -> Pacific Ocean 
8       [2,2] -> [2,3] -> [2,4] -> Atlantic Ocean
9[3,0]: [3,0] -> Pacific Ocean 
10       [3,0] -> [4,0] -> Atlantic Ocean
11[3,1]: [3,1] -> [3,0] -> Pacific Ocean 
12       [3,1] -> [4,1] -> Atlantic Ocean
13[4,0]: [4,0] -> Pacific Ocean 
14      [4,0] -> Atlantic Ocean

Note that there are other possible paths for these cells to flow to the Pacific and Atlantic oceans.

Example 2:
Input: heights = [[1]]
Output: [[0,0]]
Explanation: The water can flow from the only cell to the Pacific and Atlantic oceans.

Constraints:

• m == heights.length
• n == heights[r].length
• 1 <= m, n <= 200
• 0 <= heights[r][c] <= 105

The best way to approach this question is to traverse from the outside cells into the inner cells. Both BFS and DFS will produce an efficient implementation. Below, we will to show a recusive dfs approach to this problem. To find the cells that will flow to both oceans, we will recursively find the cells that can be reached by the Pacific Ocean, and the cells reached by the Atlantic Ocean. Finally, we find the overlapping cells that can be reached by both oceans.

Implementation

1def pacificAtlantic(self, heights: List[List[int]]) -> List[List[int]]:
2    m = len(heights)
3    n = len(heights[0])
4    pacific = [[False for _ in range(n)] for _ in range(m)]
5    atlantic = [[False for _ in range(n)] for _ in range(m)]
6    res = []
7    
8    dirs = [(0,1), (0,-1), (1,0), (-1,0)]
9    def get_neighbours(x, y):
10        neighbours = []
11        for dirx, diry in dirs:
12            newx, newy = x+dirx, y+diry
13            if 0 <= newx < m and 0 <= newy < n:
14                neighbours.append((newx, newy))
15        return neighbours
16    
17    def dfs(x, y, ocean):
18        if ocean[x][y]: return
19        ocean[x][y] = True
20        for newx, newy in get_neighbours(x,y):
21            if heights[newx][newy] >= heights[x][y]:
22                dfs(newx, newy, ocean)
23
24    for x in range(m):
25        dfs(x, 0, pacific)
26        dfs(x, n-1, atlantic)
27    for y in range(n):
28        dfs(0, y, pacific)
29        dfs(m-1, y, atlantic)
30
31    for x in range(m):
32        for y in range(n):
33            if pacific[x][y] and atlantic[x][y]:
34                res.append([x,y])
35    return res